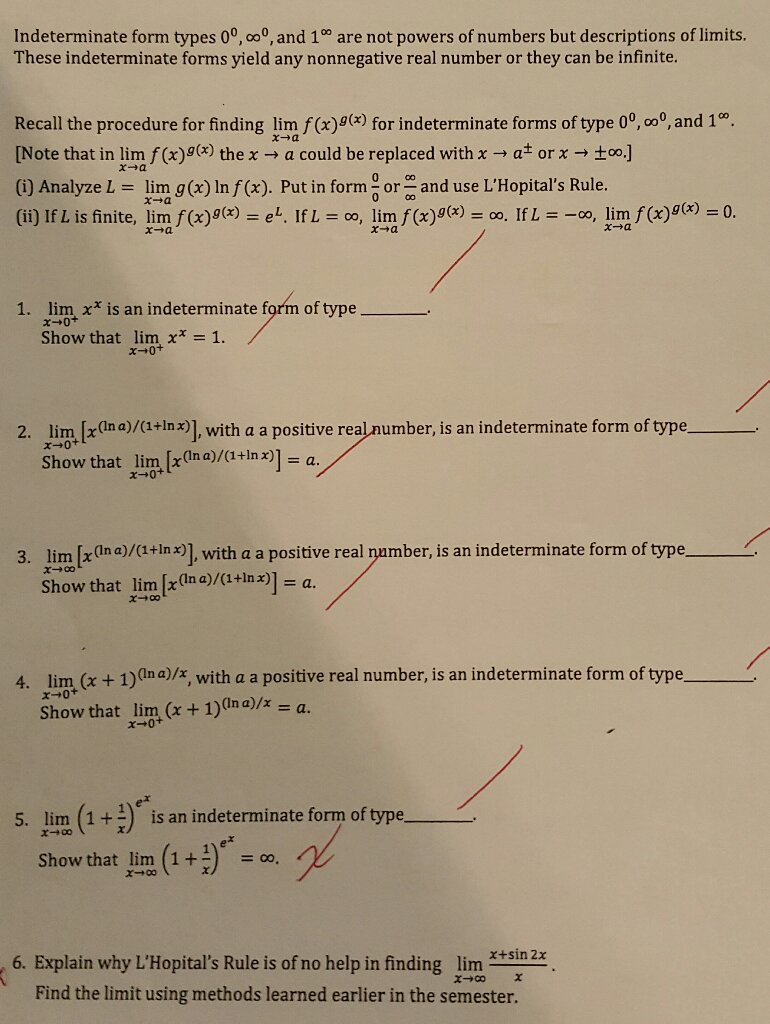0 Infinity Indeterminate Form - If $f(x)$ approaches $0$ from above, then the limit of $\frac{p(x)}{f(x)}$ is infinity. You can usually solve a limit of the form $0 \cdot \infty$ using l'hospital's rule by introducing a fraction. L’hospital’s rule works great on the two indeterminate forms 0/0 and \({{ \pm \,\infty }}/{{ \pm \,\infty }}\;\). The process of finding the. If $f(x)$ approaches $0$ from below, then the. An indeterminate form is an expression formed with two of 1, 0, and infinity, and its value cannot be de determined. Specifically, if $f(x) \to 0$ and $g(x).
L’hospital’s rule works great on the two indeterminate forms 0/0 and \({{ \pm \,\infty }}/{{ \pm \,\infty }}\;\). If $f(x)$ approaches $0$ from below, then the. An indeterminate form is an expression formed with two of 1, 0, and infinity, and its value cannot be de determined. If $f(x)$ approaches $0$ from above, then the limit of $\frac{p(x)}{f(x)}$ is infinity. Specifically, if $f(x) \to 0$ and $g(x). The process of finding the. You can usually solve a limit of the form $0 \cdot \infty$ using l'hospital's rule by introducing a fraction.
An indeterminate form is an expression formed with two of 1, 0, and infinity, and its value cannot be de determined. Specifically, if $f(x) \to 0$ and $g(x). The process of finding the. You can usually solve a limit of the form $0 \cdot \infty$ using l'hospital's rule by introducing a fraction. L’hospital’s rule works great on the two indeterminate forms 0/0 and \({{ \pm \,\infty }}/{{ \pm \,\infty }}\;\). If $f(x)$ approaches $0$ from below, then the. If $f(x)$ approaches $0$ from above, then the limit of $\frac{p(x)}{f(x)}$ is infinity.
Indeterminate form types 0^0, infinity^0, and
The process of finding the. L’hospital’s rule works great on the two indeterminate forms 0/0 and \({{ \pm \,\infty }}/{{ \pm \,\infty }}\;\). If $f(x)$ approaches $0$ from below, then the. If $f(x)$ approaches $0$ from above, then the limit of $\frac{p(x)}{f(x)}$ is infinity. An indeterminate form is an expression formed with two of 1, 0, and infinity, and its.
Indeterminate Form 0 to 0 YouTube
The process of finding the. You can usually solve a limit of the form $0 \cdot \infty$ using l'hospital's rule by introducing a fraction. L’hospital’s rule works great on the two indeterminate forms 0/0 and \({{ \pm \,\infty }}/{{ \pm \,\infty }}\;\). An indeterminate form is an expression formed with two of 1, 0, and infinity, and its value cannot.
Solved Show that 0 infinity is not an indeterminate form by
Specifically, if $f(x) \to 0$ and $g(x). If $f(x)$ approaches $0$ from above, then the limit of $\frac{p(x)}{f(x)}$ is infinity. You can usually solve a limit of the form $0 \cdot \infty$ using l'hospital's rule by introducing a fraction. If $f(x)$ approaches $0$ from below, then the. The process of finding the.
Indeterminate Form Infinity Infinity YouTube
Specifically, if $f(x) \to 0$ and $g(x). You can usually solve a limit of the form $0 \cdot \infty$ using l'hospital's rule by introducing a fraction. L’hospital’s rule works great on the two indeterminate forms 0/0 and \({{ \pm \,\infty }}/{{ \pm \,\infty }}\;\). The process of finding the. If $f(x)$ approaches $0$ from below, then the.
Calculus Indeterminate Forms YouTube
L’hospital’s rule works great on the two indeterminate forms 0/0 and \({{ \pm \,\infty }}/{{ \pm \,\infty }}\;\). Specifically, if $f(x) \to 0$ and $g(x). An indeterminate form is an expression formed with two of 1, 0, and infinity, and its value cannot be de determined. You can usually solve a limit of the form $0 \cdot \infty$ using l'hospital's.
Finding Indeterminate Limits L'Hôpital's Rule 0/0, infinity
An indeterminate form is an expression formed with two of 1, 0, and infinity, and its value cannot be de determined. The process of finding the. If $f(x)$ approaches $0$ from below, then the. Specifically, if $f(x) \to 0$ and $g(x). L’hospital’s rule works great on the two indeterminate forms 0/0 and \({{ \pm \,\infty }}/{{ \pm \,\infty }}\;\).
Indeterminate form 0*infinity example Math, Calculus, Limits, 0
If $f(x)$ approaches $0$ from below, then the. If $f(x)$ approaches $0$ from above, then the limit of $\frac{p(x)}{f(x)}$ is infinity. The process of finding the. You can usually solve a limit of the form $0 \cdot \infty$ using l'hospital's rule by introducing a fraction. L’hospital’s rule works great on the two indeterminate forms 0/0 and \({{ \pm \,\infty }}/{{.
6.9 Indeterminate form ZERO times INFINITY YouTube
An indeterminate form is an expression formed with two of 1, 0, and infinity, and its value cannot be de determined. If $f(x)$ approaches $0$ from below, then the. L’hospital’s rule works great on the two indeterminate forms 0/0 and \({{ \pm \,\infty }}/{{ \pm \,\infty }}\;\). The process of finding the. You can usually solve a limit of the.
Indeterminate form 0 times INFINITY YouTube
An indeterminate form is an expression formed with two of 1, 0, and infinity, and its value cannot be de determined. Specifically, if $f(x) \to 0$ and $g(x). If $f(x)$ approaches $0$ from below, then the. You can usually solve a limit of the form $0 \cdot \infty$ using l'hospital's rule by introducing a fraction. L’hospital’s rule works great on.
What Is Infinity Multiplied By 0
If $f(x)$ approaches $0$ from above, then the limit of $\frac{p(x)}{f(x)}$ is infinity. You can usually solve a limit of the form $0 \cdot \infty$ using l'hospital's rule by introducing a fraction. An indeterminate form is an expression formed with two of 1, 0, and infinity, and its value cannot be de determined. L’hospital’s rule works great on the two.
L’hospital’s Rule Works Great On The Two Indeterminate Forms 0/0 And \({{ \Pm \,\Infty }}/{{ \Pm \,\Infty }}\;\).
If $f(x)$ approaches $0$ from below, then the. If $f(x)$ approaches $0$ from above, then the limit of $\frac{p(x)}{f(x)}$ is infinity. The process of finding the. Specifically, if $f(x) \to 0$ and $g(x).
An Indeterminate Form Is An Expression Formed With Two Of 1, 0, And Infinity, And Its Value Cannot Be De Determined.
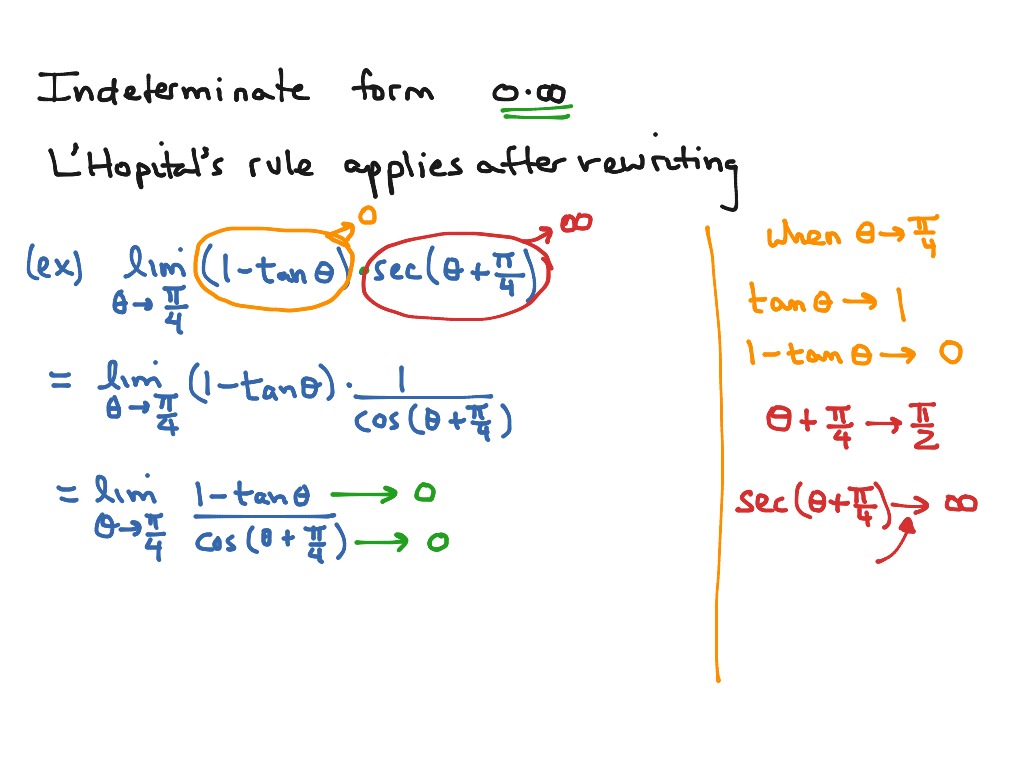
You can usually solve a limit of the form $0 \cdot \infty$ using l'hospital's rule by introducing a fraction.