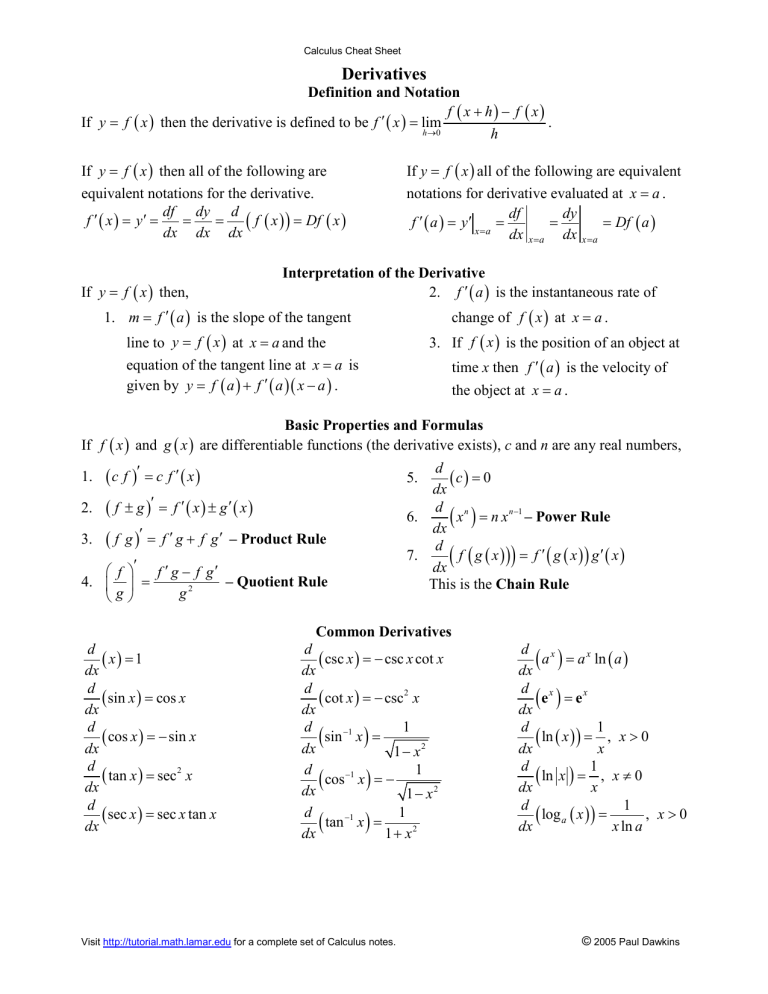
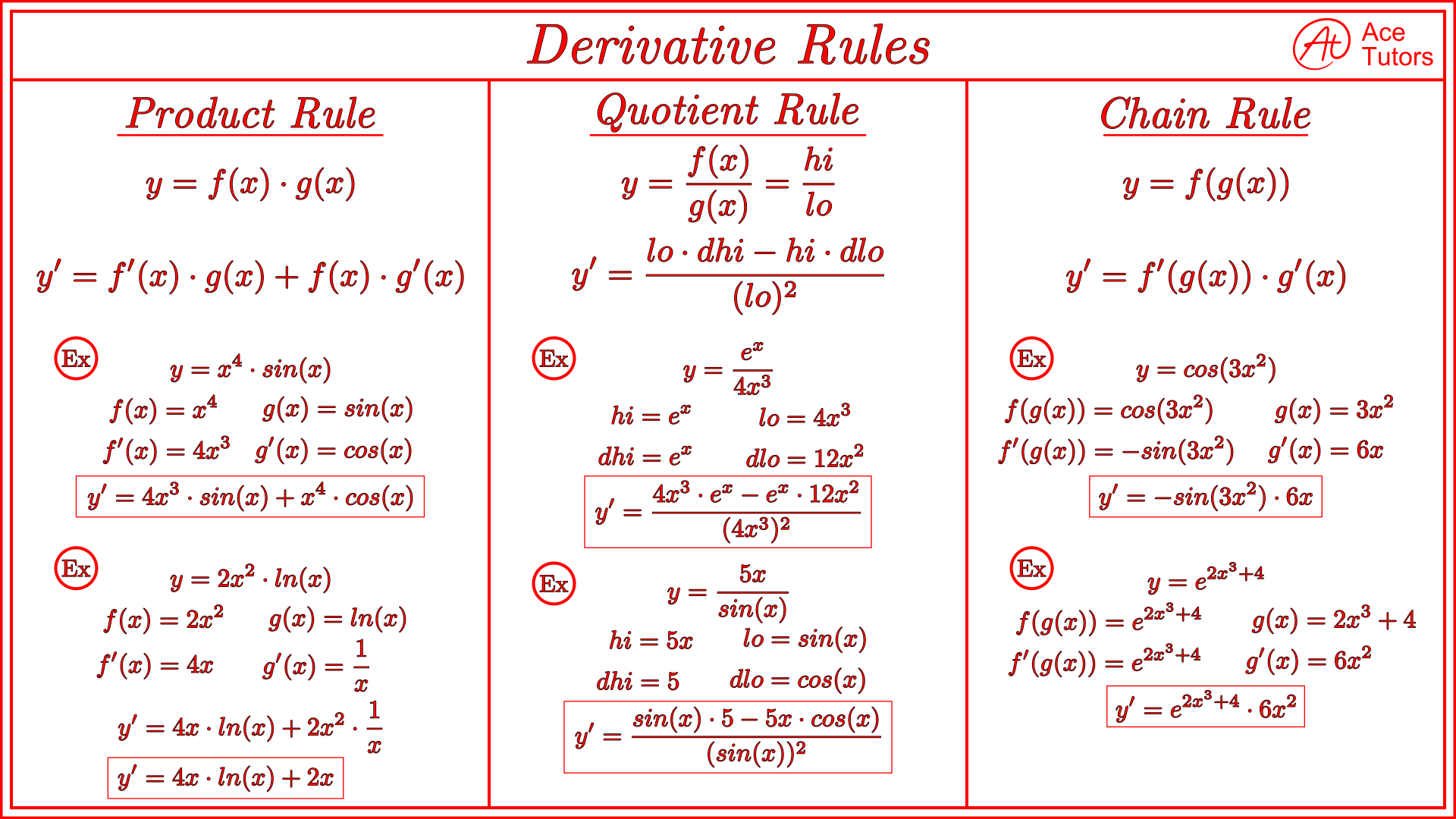
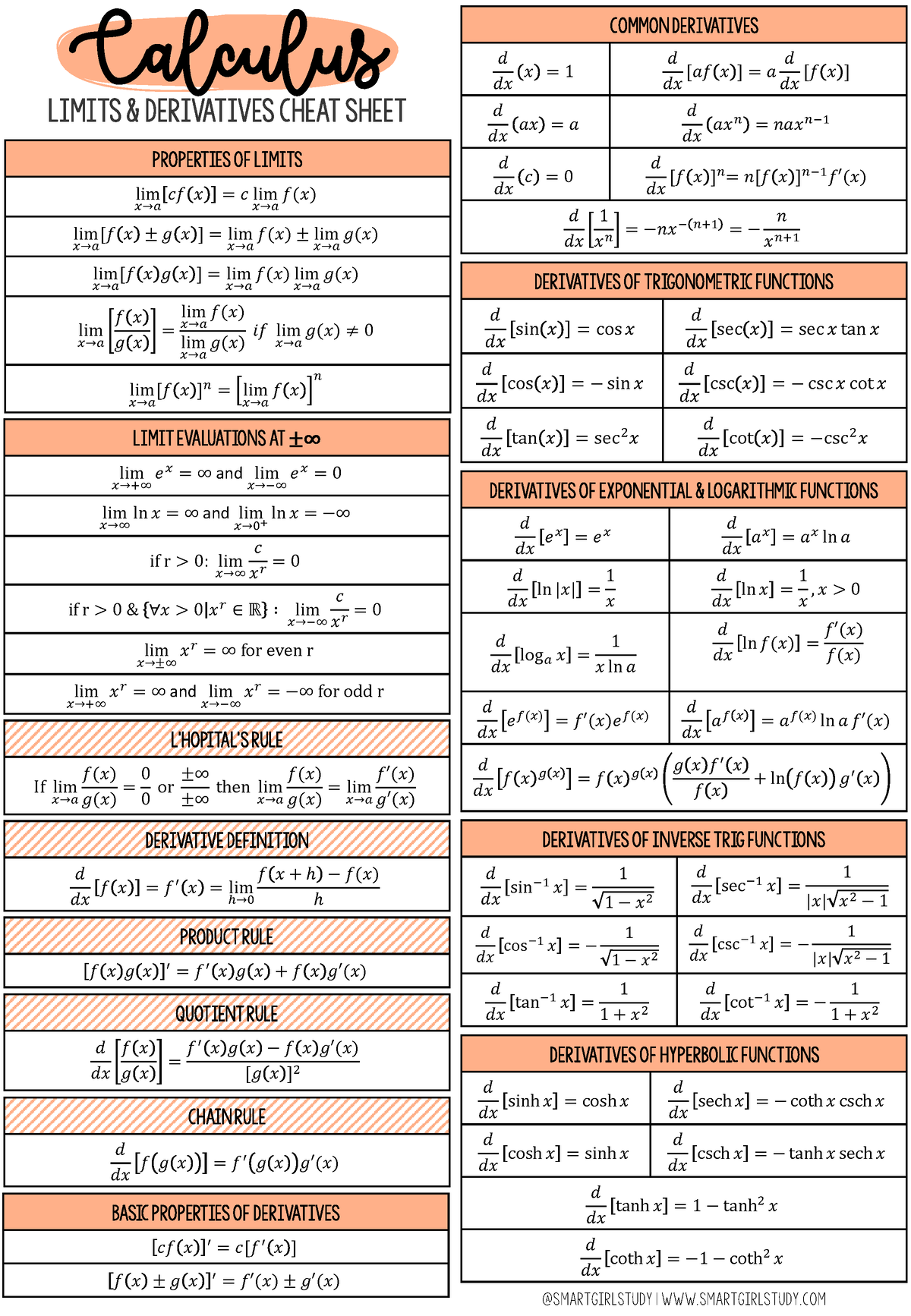
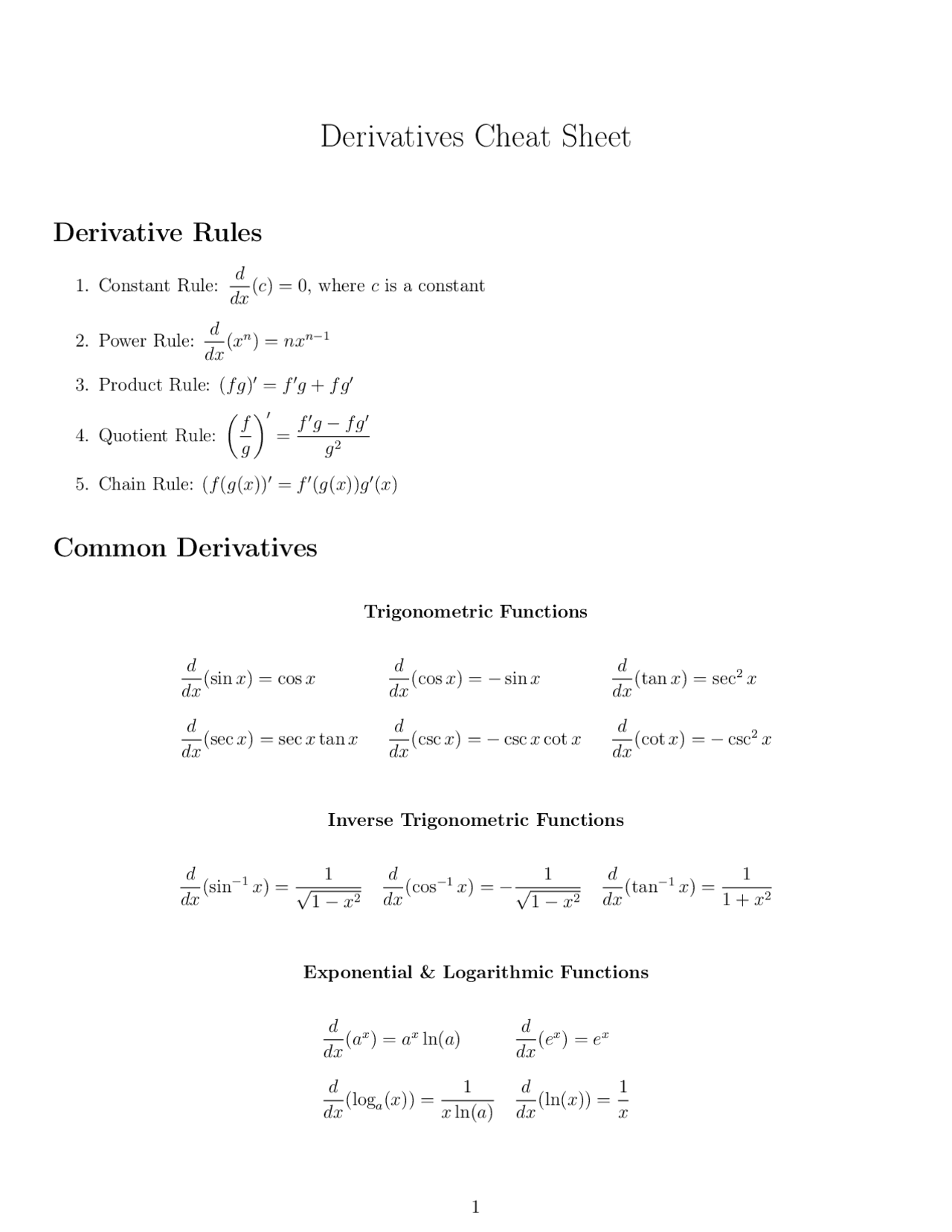
Calculus Derivative Cheat Sheet - The chain rule applied to some specific functions. \frac {d} {dx}\left (e^ {x})=e^ {x} \frac {d} {dx}\left (\log (x))=\frac {1} {x\ln (10)} \frac {d} {dx}\left (\log_ {a} (x))=\frac {1} {x\ln (a)} \frac {d}. Add on a derivative every. Write down equation relating quantities and differentiate with respect to t using implicit differentiation (i.e. ¢ f ¢¢ ( x ) = ( f ¢ ( x ) ).
Write down equation relating quantities and differentiate with respect to t using implicit differentiation (i.e. The chain rule applied to some specific functions. ¢ f ¢¢ ( x ) = ( f ¢ ( x ) ). \frac {d} {dx}\left (e^ {x})=e^ {x} \frac {d} {dx}\left (\log (x))=\frac {1} {x\ln (10)} \frac {d} {dx}\left (\log_ {a} (x))=\frac {1} {x\ln (a)} \frac {d}. Add on a derivative every.
\frac {d} {dx}\left (e^ {x})=e^ {x} \frac {d} {dx}\left (\log (x))=\frac {1} {x\ln (10)} \frac {d} {dx}\left (\log_ {a} (x))=\frac {1} {x\ln (a)} \frac {d}. Add on a derivative every. Write down equation relating quantities and differentiate with respect to t using implicit differentiation (i.e. The chain rule applied to some specific functions. ¢ f ¢¢ ( x ) = ( f ¢ ( x ) ).
Calculus Cheat Sheet Derivatives
Add on a derivative every. The chain rule applied to some specific functions. Write down equation relating quantities and differentiate with respect to t using implicit differentiation (i.e. ¢ f ¢¢ ( x ) = ( f ¢ ( x ) ). \frac {d} {dx}\left (e^ {x})=e^ {x} \frac {d} {dx}\left (\log (x))=\frac {1} {x\ln (10)} \frac {d} {dx}\left (\log_.
Matrix Derivative Cheat Sheet
The chain rule applied to some specific functions. Write down equation relating quantities and differentiate with respect to t using implicit differentiation (i.e. \frac {d} {dx}\left (e^ {x})=e^ {x} \frac {d} {dx}\left (\log (x))=\frac {1} {x\ln (10)} \frac {d} {dx}\left (\log_ {a} (x))=\frac {1} {x\ln (a)} \frac {d}. Add on a derivative every. ¢ f ¢¢ ( x ) =.
Calculus derivatives rules and limits cheat sheet eeweb Artofit
The chain rule applied to some specific functions. \frac {d} {dx}\left (e^ {x})=e^ {x} \frac {d} {dx}\left (\log (x))=\frac {1} {x\ln (10)} \frac {d} {dx}\left (\log_ {a} (x))=\frac {1} {x\ln (a)} \frac {d}. Add on a derivative every. Write down equation relating quantities and differentiate with respect to t using implicit differentiation (i.e. ¢ f ¢¢ ( x ) =.
Application Of Derivatives Calculus
Add on a derivative every. ¢ f ¢¢ ( x ) = ( f ¢ ( x ) ). The chain rule applied to some specific functions. Write down equation relating quantities and differentiate with respect to t using implicit differentiation (i.e. \frac {d} {dx}\left (e^ {x})=e^ {x} \frac {d} {dx}\left (\log (x))=\frac {1} {x\ln (10)} \frac {d} {dx}\left (\log_.
Calculus DERIVATIVES Study Outline 1 Page Cheat Sheet Digital File Only
Add on a derivative every. \frac {d} {dx}\left (e^ {x})=e^ {x} \frac {d} {dx}\left (\log (x))=\frac {1} {x\ln (10)} \frac {d} {dx}\left (\log_ {a} (x))=\frac {1} {x\ln (a)} \frac {d}. ¢ f ¢¢ ( x ) = ( f ¢ ( x ) ). The chain rule applied to some specific functions. Write down equation relating quantities and differentiate with.
Cheat Sheet Calculus Maxima And Minima Derivative
Add on a derivative every. ¢ f ¢¢ ( x ) = ( f ¢ ( x ) ). Write down equation relating quantities and differentiate with respect to t using implicit differentiation (i.e. \frac {d} {dx}\left (e^ {x})=e^ {x} \frac {d} {dx}\left (\log (x))=\frac {1} {x\ln (10)} \frac {d} {dx}\left (\log_ {a} (x))=\frac {1} {x\ln (a)} \frac {d}. The.
Calculus DERIVATIVES Study Outline 1 Page Cheat Sheet Digital File Only
\frac {d} {dx}\left (e^ {x})=e^ {x} \frac {d} {dx}\left (\log (x))=\frac {1} {x\ln (10)} \frac {d} {dx}\left (\log_ {a} (x))=\frac {1} {x\ln (a)} \frac {d}. The chain rule applied to some specific functions. Add on a derivative every. ¢ f ¢¢ ( x ) = ( f ¢ ( x ) ). Write down equation relating quantities and differentiate with.
Calculus_Cheat_Sheet_All_Reduced.pdf Maxima And Minima Derivative
¢ f ¢¢ ( x ) = ( f ¢ ( x ) ). Write down equation relating quantities and differentiate with respect to t using implicit differentiation (i.e. The chain rule applied to some specific functions. \frac {d} {dx}\left (e^ {x})=e^ {x} \frac {d} {dx}\left (\log (x))=\frac {1} {x\ln (10)} \frac {d} {dx}\left (\log_ {a} (x))=\frac {1} {x\ln (a)}.
Calculus Cheat Sheet i dont know la Limits & Derivatives Cheat
The chain rule applied to some specific functions. ¢ f ¢¢ ( x ) = ( f ¢ ( x ) ). Write down equation relating quantities and differentiate with respect to t using implicit differentiation (i.e. Add on a derivative every. \frac {d} {dx}\left (e^ {x})=e^ {x} \frac {d} {dx}\left (\log (x))=\frac {1} {x\ln (10)} \frac {d} {dx}\left (\log_.
Derivative cheat sheet Cheat Sheet Mathematical Analysis Docsity
The chain rule applied to some specific functions. \frac {d} {dx}\left (e^ {x})=e^ {x} \frac {d} {dx}\left (\log (x))=\frac {1} {x\ln (10)} \frac {d} {dx}\left (\log_ {a} (x))=\frac {1} {x\ln (a)} \frac {d}. Add on a derivative every. ¢ f ¢¢ ( x ) = ( f ¢ ( x ) ). Write down equation relating quantities and differentiate with.
\Frac {D} {Dx}\Left (E^ {X})=E^ {X} \Frac {D} {Dx}\Left (\Log (X))=\Frac {1} {X\Ln (10)} \Frac {D} {Dx}\Left (\Log_ {A} (X))=\Frac {1} {X\Ln (A)} \Frac {D}.
The chain rule applied to some specific functions. ¢ f ¢¢ ( x ) = ( f ¢ ( x ) ). Add on a derivative every. Write down equation relating quantities and differentiate with respect to t using implicit differentiation (i.e.