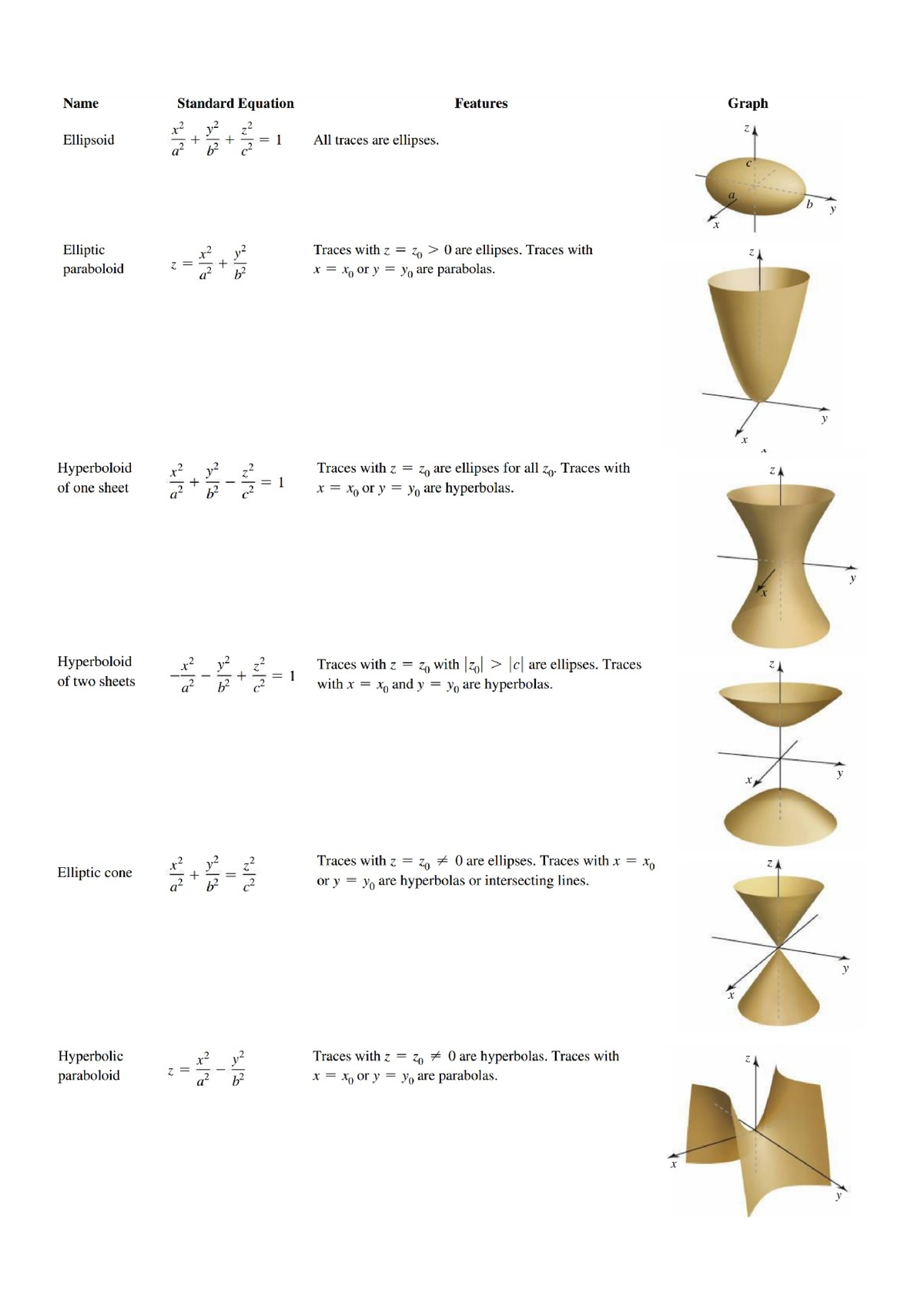
Quadric Surfaces Cheat Sheet - A quadratic surface intersects every plane in a (proper or. Quadric surface examples example 1 (12.6.30). Quadric surfaces are the graphs of any equation that can be put into the general form \[a{x^2} + b{y^2} + c{z^2} + dxy + exz + fyz + gx + hy + iz + j = 0\] where \(a\),. Use traces to draw the intersections of quadric surfaces with the coordinate planes. If a quadric surface is symmetric about a different axis, its equation changes accordingly. Recognize the main features of ellipsoids, paraboloids, and hyperboloids.
Quadric surface examples example 1 (12.6.30). Quadric surfaces are the graphs of any equation that can be put into the general form \[a{x^2} + b{y^2} + c{z^2} + dxy + exz + fyz + gx + hy + iz + j = 0\] where \(a\),. If a quadric surface is symmetric about a different axis, its equation changes accordingly. A quadratic surface intersects every plane in a (proper or. Recognize the main features of ellipsoids, paraboloids, and hyperboloids. Use traces to draw the intersections of quadric surfaces with the coordinate planes.
Quadric surface examples example 1 (12.6.30). If a quadric surface is symmetric about a different axis, its equation changes accordingly. Recognize the main features of ellipsoids, paraboloids, and hyperboloids. Use traces to draw the intersections of quadric surfaces with the coordinate planes. Quadric surfaces are the graphs of any equation that can be put into the general form \[a{x^2} + b{y^2} + c{z^2} + dxy + exz + fyz + gx + hy + iz + j = 0\] where \(a\),. A quadratic surface intersects every plane in a (proper or.
Quadratic Surfaces Cheat Sheet MA 26100 Studocu
Use traces to draw the intersections of quadric surfaces with the coordinate planes. Quadric surface examples example 1 (12.6.30). A quadratic surface intersects every plane in a (proper or. If a quadric surface is symmetric about a different axis, its equation changes accordingly. Quadric surfaces are the graphs of any equation that can be put into the general form \[a{x^2}.
Quadric Surfaces Part 6 Practice Identifying YouTube
Use traces to draw the intersections of quadric surfaces with the coordinate planes. Quadric surfaces are the graphs of any equation that can be put into the general form \[a{x^2} + b{y^2} + c{z^2} + dxy + exz + fyz + gx + hy + iz + j = 0\] where \(a\),. Recognize the main features of ellipsoids, paraboloids, and.
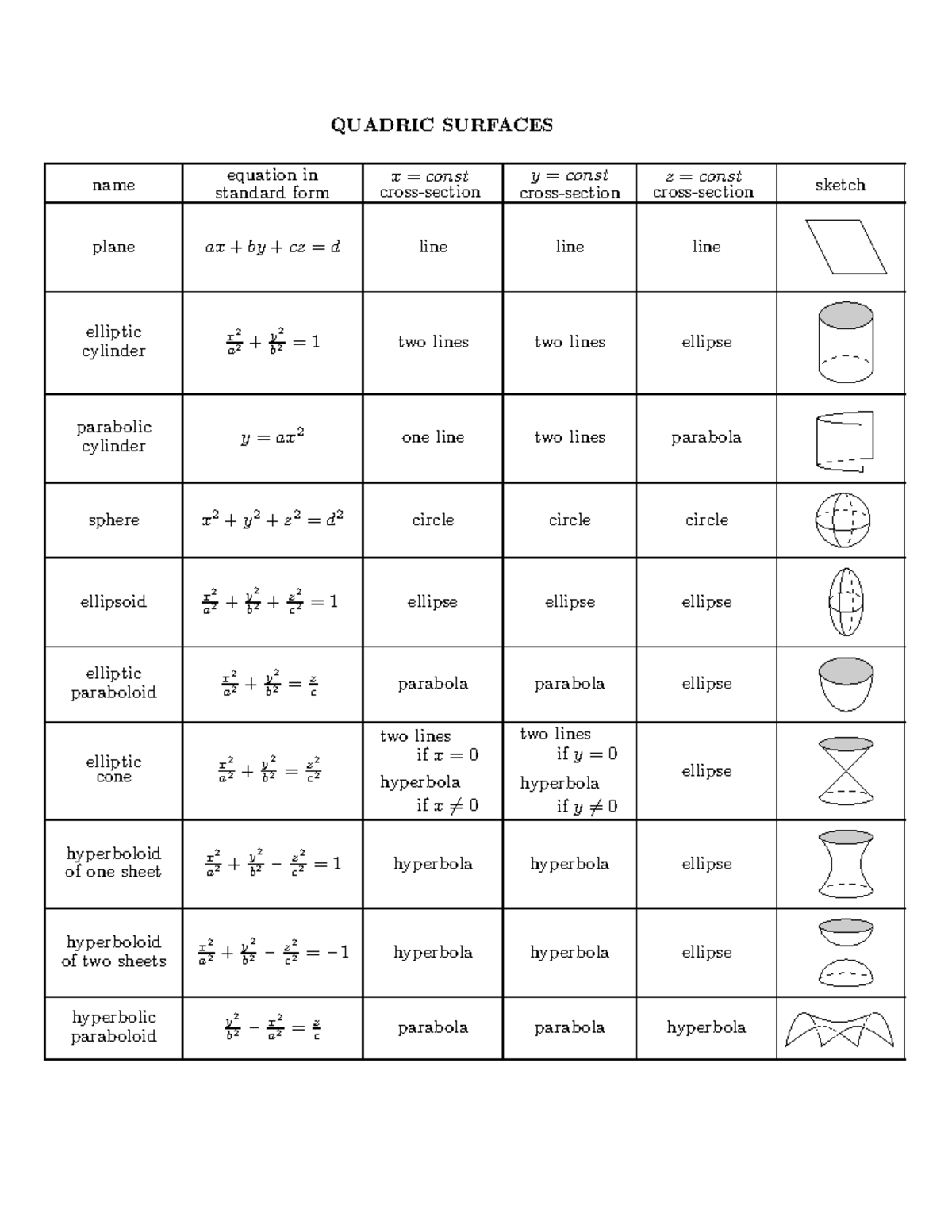
M220 Quadric Surfaces Info Sheets QUADRIC SURFACES name equation in
Quadric surfaces are the graphs of any equation that can be put into the general form \[a{x^2} + b{y^2} + c{z^2} + dxy + exz + fyz + gx + hy + iz + j = 0\] where \(a\),. A quadratic surface intersects every plane in a (proper or. Use traces to draw the intersections of quadric surfaces with the.
Mary's Notes Learn physics, How to memorize things, Notes
Use traces to draw the intersections of quadric surfaces with the coordinate planes. A quadratic surface intersects every plane in a (proper or. Quadric surfaces are the graphs of any equation that can be put into the general form \[a{x^2} + b{y^2} + c{z^2} + dxy + exz + fyz + gx + hy + iz + j = 0\].
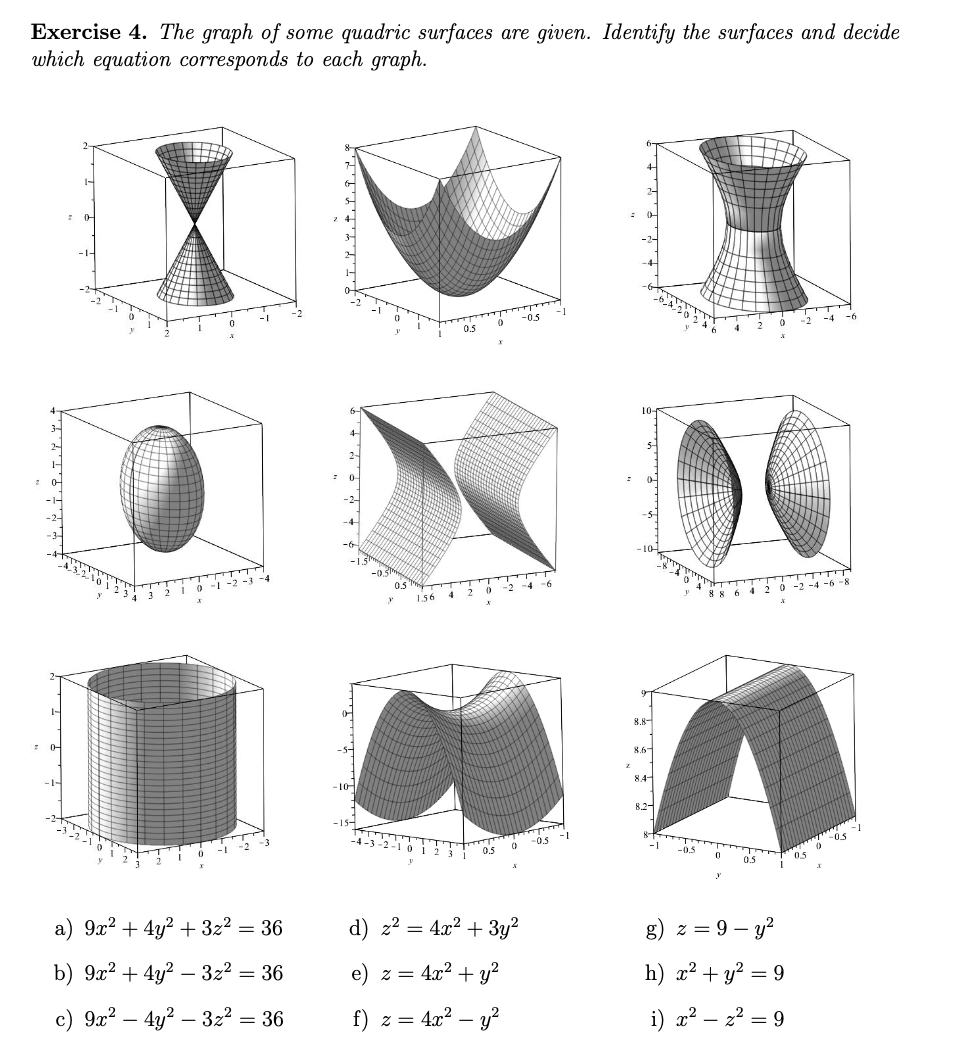
Solved Exercise 4. The graph of some quadric surfaces are
Recognize the main features of ellipsoids, paraboloids, and hyperboloids. If a quadric surface is symmetric about a different axis, its equation changes accordingly. Quadric surfaces are the graphs of any equation that can be put into the general form \[a{x^2} + b{y^2} + c{z^2} + dxy + exz + fyz + gx + hy + iz + j = 0\].
Calc 3 Quadratic Surfaces Formula to Know YouTube
Quadric surface examples example 1 (12.6.30). If a quadric surface is symmetric about a different axis, its equation changes accordingly. Recognize the main features of ellipsoids, paraboloids, and hyperboloids. Use traces to draw the intersections of quadric surfaces with the coordinate planes. Quadric surfaces are the graphs of any equation that can be put into the general form \[a{x^2} +.
CIII_E_Graphs of Quadric Surfaces
Quadric surfaces are the graphs of any equation that can be put into the general form \[a{x^2} + b{y^2} + c{z^2} + dxy + exz + fyz + gx + hy + iz + j = 0\] where \(a\),. If a quadric surface is symmetric about a different axis, its equation changes accordingly. Quadric surface examples example 1 (12.6.30). Use.
Quadric surfaces MATH 277
Quadric surfaces are the graphs of any equation that can be put into the general form \[a{x^2} + b{y^2} + c{z^2} + dxy + exz + fyz + gx + hy + iz + j = 0\] where \(a\),. Recognize the main features of ellipsoids, paraboloids, and hyperboloids. Quadric surface examples example 1 (12.6.30). Use traces to draw the intersections.
11.6 Quadric Surfaces Mathematics LibreTexts
A quadratic surface intersects every plane in a (proper or. Use traces to draw the intersections of quadric surfaces with the coordinate planes. If a quadric surface is symmetric about a different axis, its equation changes accordingly. Quadric surface examples example 1 (12.6.30). Quadric surfaces are the graphs of any equation that can be put into the general form \[a{x^2}.
![[PDF] TypeConstrained Direct Fitting of Quadric Surfaces Semantic](https://d3i71xaburhd42.cloudfront.net/44c43edcab0fca85ad5184866af596febea31b7b/3-Figure2-1.png)
[PDF] TypeConstrained Direct Fitting of Quadric Surfaces Semantic
A quadratic surface intersects every plane in a (proper or. If a quadric surface is symmetric about a different axis, its equation changes accordingly. Quadric surfaces are the graphs of any equation that can be put into the general form \[a{x^2} + b{y^2} + c{z^2} + dxy + exz + fyz + gx + hy + iz + j =.
Use Traces To Draw The Intersections Of Quadric Surfaces With The Coordinate Planes.
A quadratic surface intersects every plane in a (proper or. If a quadric surface is symmetric about a different axis, its equation changes accordingly. Quadric surfaces are the graphs of any equation that can be put into the general form \[a{x^2} + b{y^2} + c{z^2} + dxy + exz + fyz + gx + hy + iz + j = 0\] where \(a\),. Recognize the main features of ellipsoids, paraboloids, and hyperboloids.