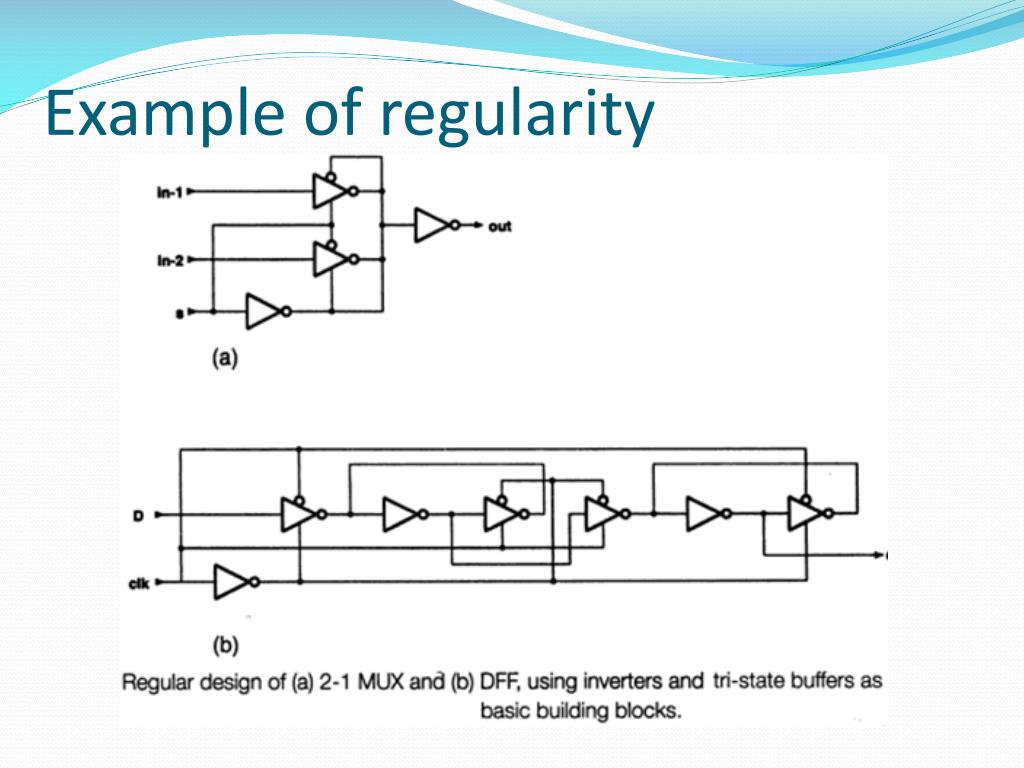
Regularity In Math Examples - Very recently, a new theory of “regularity structures” was introduced [hai14], unifying various flavours of the theory of (controlled) rough paths (including. $m$ is regular if, for any $a\in\mathcal{a}$, the measure of $a$ equals the infimum of measures. This includes all rational functions, which are built up from combinations of the function x with. A regularity condition is essentially just a requirement that whatever structure you are studying isn't too poorly behaved. While many problems or tasks do require students to use a combination of. Look for and express regularity in repeated reasoning. The regularity a solution can inherit depends on the properties of the problem, i.e., the smoothness of a domain boundary, the. The standard definition of regularity goes like this: Every elementary function is continuous.
The standard definition of regularity goes like this: $m$ is regular if, for any $a\in\mathcal{a}$, the measure of $a$ equals the infimum of measures. Every elementary function is continuous. Very recently, a new theory of “regularity structures” was introduced [hai14], unifying various flavours of the theory of (controlled) rough paths (including. While many problems or tasks do require students to use a combination of. A regularity condition is essentially just a requirement that whatever structure you are studying isn't too poorly behaved. Look for and express regularity in repeated reasoning. The regularity a solution can inherit depends on the properties of the problem, i.e., the smoothness of a domain boundary, the. This includes all rational functions, which are built up from combinations of the function x with.
Every elementary function is continuous. A regularity condition is essentially just a requirement that whatever structure you are studying isn't too poorly behaved. This includes all rational functions, which are built up from combinations of the function x with. The regularity a solution can inherit depends on the properties of the problem, i.e., the smoothness of a domain boundary, the. Very recently, a new theory of “regularity structures” was introduced [hai14], unifying various flavours of the theory of (controlled) rough paths (including. $m$ is regular if, for any $a\in\mathcal{a}$, the measure of $a$ equals the infimum of measures. While many problems or tasks do require students to use a combination of. The standard definition of regularity goes like this: Look for and express regularity in repeated reasoning.
The Common Fixed Point Theorems for Asymptotic Regularity on bMetric
A regularity condition is essentially just a requirement that whatever structure you are studying isn't too poorly behaved. $m$ is regular if, for any $a\in\mathcal{a}$, the measure of $a$ equals the infimum of measures. Look for and express regularity in repeated reasoning. This includes all rational functions, which are built up from combinations of the function x with. Every elementary.
Regularity Regularity in Language Regularity in Pragmatics YouTube
The regularity a solution can inherit depends on the properties of the problem, i.e., the smoothness of a domain boundary, the. Very recently, a new theory of “regularity structures” was introduced [hai14], unifying various flavours of the theory of (controlled) rough paths (including. While many problems or tasks do require students to use a combination of. The standard definition of.
Look for and Express Regularity in Repeated Reasoning… in Math!
The standard definition of regularity goes like this: While many problems or tasks do require students to use a combination of. Every elementary function is continuous. Look for and express regularity in repeated reasoning. The regularity a solution can inherit depends on the properties of the problem, i.e., the smoothness of a domain boundary, the.
Pattern and Regularities Mathematics in the Modern World YouTube
$m$ is regular if, for any $a\in\mathcal{a}$, the measure of $a$ equals the infimum of measures. While many problems or tasks do require students to use a combination of. Look for and express regularity in repeated reasoning. A regularity condition is essentially just a requirement that whatever structure you are studying isn't too poorly behaved. This includes all rational functions,.
(PDF) {\mathscr {A}}quasiconvexity and partial regularity
$m$ is regular if, for any $a\in\mathcal{a}$, the measure of $a$ equals the infimum of measures. The regularity a solution can inherit depends on the properties of the problem, i.e., the smoothness of a domain boundary, the. A regularity condition is essentially just a requirement that whatever structure you are studying isn't too poorly behaved. While many problems or tasks.
PPT Chapter 1 PowerPoint Presentation, free download ID1587802
While many problems or tasks do require students to use a combination of. The regularity a solution can inherit depends on the properties of the problem, i.e., the smoothness of a domain boundary, the. This includes all rational functions, which are built up from combinations of the function x with. $m$ is regular if, for any $a\in\mathcal{a}$, the measure of.
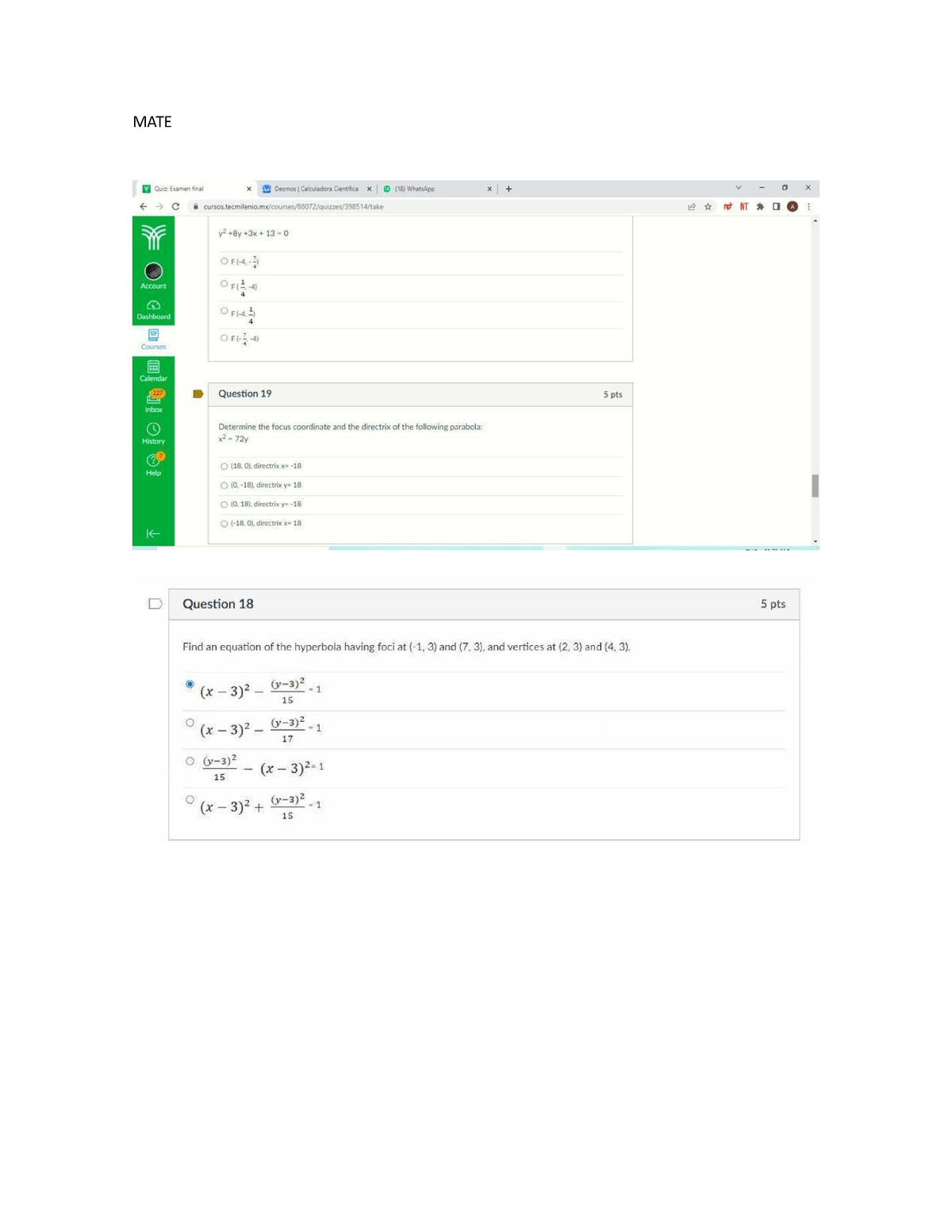
MATE Examen Final EXAM Math III regularity and repetition MATE
While many problems or tasks do require students to use a combination of. The regularity a solution can inherit depends on the properties of the problem, i.e., the smoothness of a domain boundary, the. A regularity condition is essentially just a requirement that whatever structure you are studying isn't too poorly behaved. Very recently, a new theory of “regularity structures”.
Regularity form OHM VITAL
Every elementary function is continuous. While many problems or tasks do require students to use a combination of. The standard definition of regularity goes like this: The regularity a solution can inherit depends on the properties of the problem, i.e., the smoothness of a domain boundary, the. Very recently, a new theory of “regularity structures” was introduced [hai14], unifying various.
Global regularity for systems with symmetric gradients
Very recently, a new theory of “regularity structures” was introduced [hai14], unifying various flavours of the theory of (controlled) rough paths (including. The regularity a solution can inherit depends on the properties of the problem, i.e., the smoothness of a domain boundary, the. Every elementary function is continuous. This includes all rational functions, which are built up from combinations of.
(PDF) A regularity theory for an initial value problem with a time
Very recently, a new theory of “regularity structures” was introduced [hai14], unifying various flavours of the theory of (controlled) rough paths (including. Every elementary function is continuous. While many problems or tasks do require students to use a combination of. The standard definition of regularity goes like this: This includes all rational functions, which are built up from combinations of.
$M$ Is Regular If, For Any $A\In\Mathcal{A}$, The Measure Of $A$ Equals The Infimum Of Measures.
While many problems or tasks do require students to use a combination of. Very recently, a new theory of “regularity structures” was introduced [hai14], unifying various flavours of the theory of (controlled) rough paths (including. A regularity condition is essentially just a requirement that whatever structure you are studying isn't too poorly behaved. The regularity a solution can inherit depends on the properties of the problem, i.e., the smoothness of a domain boundary, the.
This Includes All Rational Functions, Which Are Built Up From Combinations Of The Function X With.
Every elementary function is continuous. Look for and express regularity in repeated reasoning. The standard definition of regularity goes like this: